
Even having introduced coordinates and verifying, I still find it very mysterious. Verifying the motion is indeed elliptical.īut I find this pretty unsatisfactory until introducing coordinates, I had no reason to believe that an ellipse should be created.
#Trammel of archimedes free
If you're willing to set up shop and start giving coordinates to the end that draws the ellipse (letting $a$ be the length of the rod, $b$ the distance from interior anchor to free end), it's not too hard to see thatĪnd so in turn $$\frac = 1,$$

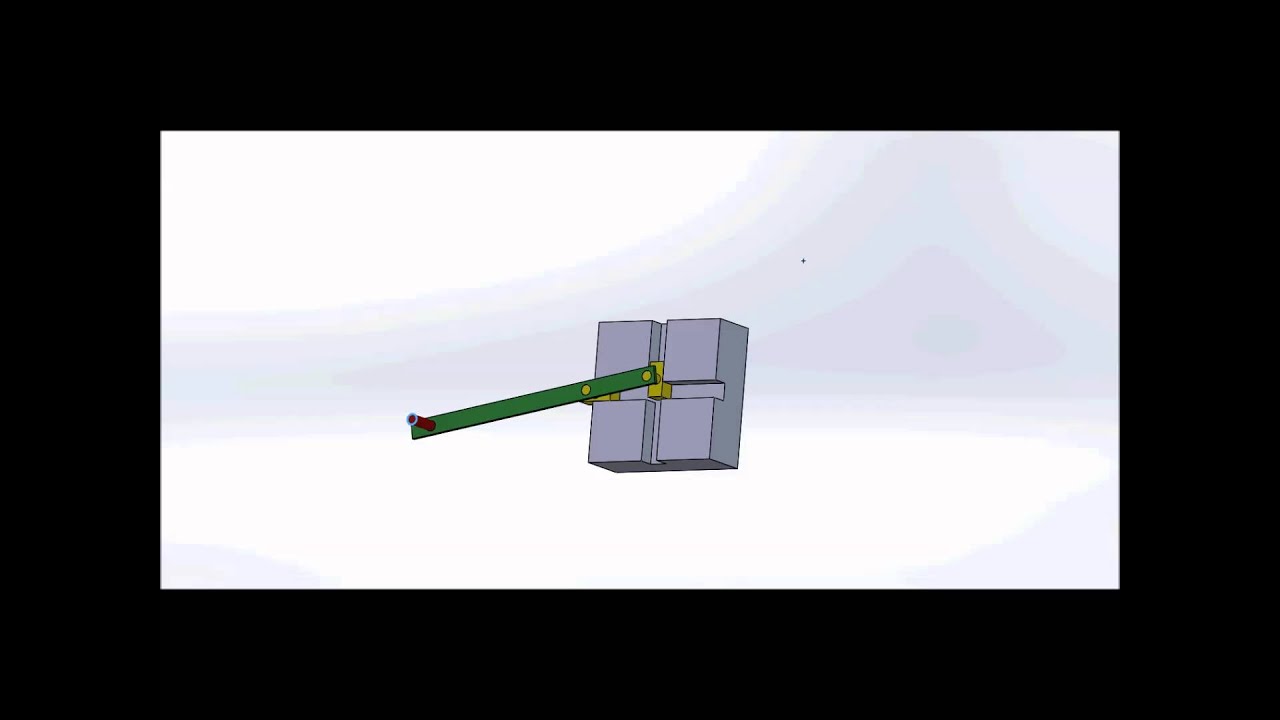
The non-anchored end (black square) can move freely, tracing an ellipse, as the sliders move along perpendicular axes - the end anchor along the minor axis, the interior anchor the major axis. I find this quite surprising, and would like to get to the bottom of things.Įssentially, a rod (black line in animation) is anchored to two sliders (in blue): One at an end, the other somewhere in the middle. gif is the one used as header of this post.) The area of the Astroid in (m2) is determined by this equation, where a is the radius of the rolling circle.Īs always, you can try using fxSolver, with the Astroid formula.Here's a diagram of the device I mean, hard at work drawing an ellipse. An astroid created by a circle rolling inside a circle of radius “a” will have an area depended on radius “a” (A very comprehensive. It is nearly identical in form to the evolute of an ellipse. The curve has a variety of names, including tetracuspid, cubocycloid, and paracycle. The astroid is a real locus of a plane algebraic curve of genus zero. An astroid is a particular mathematical curve: a hypocycloid with four cusps. The envelope of the moving bar of the Trammel of Archimedes, is the astroid. Usually the distances a and b are adjustable, so that the size and shape of the ellipse can be varied. An ellipsograph has the appropriate instrument (pencil, knife, router, etc.) attached to the rod. An ellipsograph is a trammel of Archimedes intended to draw, cut, or machine ellipses, e.g. The semi-axes a and b of the ellipse are the distances between the end of the rod and the two pivots. As the shuttles move back and forth, each along its channel, the end of the rod moves in an elliptical path. It consists of two shuttles which are confined (“trammelled”) to perpendicular channels or rails, and a rod which is attached to the shuttles by pivots at fixed positions along the rod. It is a mechanism that traces out an ellipse. The word that he was crying naked in the streets when he invented a method for determining the volume of an object with an irregular shape.Īs we mentioned before he invented the “ Trammel of his“ (the two.
The majority of people know him from his famous “ Eureka“. He was also one of the first to apply mathematics to physical phenomena, founding hydrostatics and statics, including an explanation of the principle of the lever. He has more mathematical achievements under his name, such as deriving an accurate approximation of pi, defining and investigating the spiral bearing his name, and creating a system using exponentiation for expressing very large numbers. He is considered one of the greatest mathematicians of all time, and he anticipated modern calculus and analysis by applying concepts of infinitesimals and the method of exhaustion to derive and rigorously prove a range of geometrical theorems, including the area of a circle, the surface area and volume of a sphere, and the trammel of Archimedes. He is regarded as one of the leading scientists in classical antiquity beside the fact that we know very little about his life. Archimedes of Syracuse was an Ancient Greek mathematician, physicist, engineer, inventor, and astronomer.


 0 kommentar(er)
0 kommentar(er)
